Behaviors of Metal-based Reactive Fragments Penetrating Spaced Aluminum Targets

ZHOU Sheng; ZHANG Jiahao; YU Qingbo

doi:10.12382/bgxb.2022.0232

Acta Armamentarii ›› 2023, Vol. 44 ›› Issue (8) : 2263-2272. DOI: 10.12382/bgxb.2022.0232

Behaviors of Metal-based Reactive Fragments Penetrating Spaced Aluminum Targets

Author information +

History +

Abstract

Ballistic impact experiments are conducted on metal-based reactive fragments impacting spaced targets to investigate the post-target debris cloud and damage effect behaviors of the reactive fragments, and to reveal the mechanism of their penetration. By observing the perforation mode of spaced target and the action behavior of fragments, we combine the breakage theory of target penetration, energy conservation law, and the reactivation response behaviors of reactive fragments to analyze and discuss the behaviors of reactive fragments penetrating spacer aluminum targets. The results show that the front target is plugging, and the rear target mainly presents the composite mode of center penetration and debris impact due to the kinetic energy-chemical energy coupling damage of post-target debris cloud. With increasing impact velocity, the reactive of reactive fragments increases. The theoretical model of the reactive fragments’ post-target debris cloud is established, and the evolution law of debris cloud is obtained. At different impact velocities, the unit debris kinetic energy is negatively correlated with unit reaction mass at the position of the critical through aperture.

Key words

metal-based reactive fragments / spaced aluminum targets / ballistic gun experiment / debris cloud

QR code of this article

Cite this article

EndNote

Ris (Procite)

Bibtex

Download Citations

ZHOU Sheng , ZHANG Jiahao , YU Qingbo. Behaviors of Metal-based Reactive Fragments Penetrating Spaced Aluminum Targets. Acta Armamentarii. 2023, 44(8): 2263-2272 https://doi.org/10.12382/bgxb.2022.0232

0 Introduction

Active materials have become one of the hot frontier research directions in the field of efficient damage because they not only have the mechanical properties of metal materials, but also have the characteristics of reaction and energy release of energetic materials^[1⇓-3]. Fluoropolymer-based active materials, which are widely used at present, have the advantages of high energy content and good damage effect, but their density and strength are low, so they have certain limitations when used in fragment fragmentation warhead^[4⇓-6]^[7]. Because of its high density, high strength and good ignition damage effect on the target, metal-based active fragments have gradually become a hot spot in the application of fragment fragmentation warhead^[8].

The US Navy released the high-density metal-based active fragment SBIR program from the end of 2007 to the beginning of 2008, which required to achieve an active fragment density of 5~8g/cm³ and an energy content of 4.2 – 8.4 kJ/G^[9]. Based on the research progress and development trend of metal-matrix active fragments at home and abroad, Chen Jin et al concluded that when the tensile strength of metal-matrix active fragments is not less than 300MPa, the elongation at break is more than 5%, and the fragment density is not less than 7g/cm³, they can be used as fragment warhead damage elements^[10]. If the density is increased to 8~10g/cm³ and the elongation at break is increased to more than 7%, the applicability is stronger. Typical metal-based active fragments such as Ni/Al have a strength of about 370MPa and a density of about 5.5g/cm³, which can be made to reach a density of more than 7g/cm³ by adding high-density metals, and the W/Zr active fragment prepared by Liu Xiaojun et Al. Has a strength of about 1860MPa and a density of about 8.34g/cm³, but its elongation at break is only 1%, and its integrity against detonation driving is poor^[11]^[12]^[13]. At present, the related research mainly focuses on the formulation and preparation of metal-based reactive fragments, penetration capability and so on, but there is little research on the formation and damage mechanism of debris cloud after the metal-based reactive fragments penetrate the target, and the behavior of debris cloud behind the target has a significant impact on the damage effect of metal-based active fragments penetrating the spacer target^{[14⇓⇓-17]}. Therefore, it is of great significance to study the debris cloud behavior and damage mechanism behind the metal matrix reactive fragment target for the application of metal matrix reactive fragment in fragmentation warhead.

In this paper, based on the penetration experiment of spherical metal matrix reactive fragments into aluminum spacer ballistic gun, combined with the theoretical analysis of the penetration process, the debris cloud formation behind the metal matrix reactive fragments penetrating into aluminum spacer and its damage to the after-effect target are studied, the theoretical model of debris cloud formation is established, and the damage mechanism is revealed.

1 Ballistic gun test method

1.1 Experimental sample

The spherical metal-based active fragments used in the experiment were prepared from a mixture of W/Zr/Ni powders with a mass ratio of 30 ∶ 50 ∶ 20. After sintering, the density of the active fragment is about 9.91g/cm³, the mass is about 2.66 G, and the size is ϕ8 mm. The active fragment and its quasi-static compressive stress-strain curve are shown in Fig. 1. The mechanical property parameters obtained from the stress-strain curves are listed in Table 1. In Fig. 1, σ_b is the breaking strength, σ_s is the yield strength, E is the elastic modulus, and δ is the elongation at break.

Fig.1 Reactive fragments and quasi-static compressive stress-strain curves

图1 活性破片及其准静态压缩应力-应变曲线

Full size|PPT slide

Table 1 Mechanical properties of reactive fragments

表1 活性破片力学性能参数

弹性模量/GPa	屈服强度/MPa	断裂强度/MPa	断裂延伸率/%
21	1128	1568	10.1

1.2 Experimental target

The ballistic gun experimental target and its structure diagram are shown in Fig. 2. The target is a 2A12 double-layer aluminum target with a size of 300 mm × 300 mm and a thickness of 6 mm and 3 mm, respectively, and the distance between the front and rear target plates is 200 mm. The front target is an activation target, which is equivalent to a target protection structure and is used for activating the active fragment energy release reaction; The rear target is an effect target, equivalent to the internal structure of the target, which is used to verify the damage effect of active fragments.

Fig.2 Experimental target and target structure schematic

图2 实验靶标及其结构示意图

Full size|PPT slide

1.3 Experimental principle

The experimental test system and its physical photos are shown in Figure 3, which is mainly composed of a 14.5mm ballistic gun, a velocimeter, a spacer target plate, and a high-speed camera. The active fragment is loaded by the ballistic gun and hits the interval target plate after obtaining a certain speed. The initial velocity of active fragments is controlled by adjusting the propellant charge, the velocimeter is placed in front of the spacer target to measure the velocity of active fragments hitting the target, and the high-speed photography is used to record the process of active fragments hitting the target.

Fig.3 Schematic and image of the experimental test system

图3 实验测试系统及其实物照片

Full size|PPT slide

2 Experimental result

In order to study the influence of impact velocity on the damage effect, the penetration experiment of active fragments into spaced aluminum target ballistic gun at impact velocity of 700 ~ 1500m/s was carried out, and the damage area of the target was defined as the transparent part of the damage target, which was obtained by image recognition method^[18].

The typical target plate damage of reactive fragments penetrating spaced targets at different impact velocities V is shown in Table 2, and the experimental data of damage area and perforation are listed in Table 3. It can be seen from Table 2 that after the active fragments penetrate the front target at different speeds, different degrees of jet reaction traces are left on the front target; With the increase of collision velocity, the reaction marks become more prominent, which is due to the fact that with the increase of collision velocity, more active fragments participate in the reaction. By comparing the perforation data in Table 3, it is found that the perforation diameter of the active fragment decreases first and then increases with the increase of the speed when penetrating the front target. The main reason is that:Active fragments are broken and partially reacted during penetration into the front target, and the mass ratio of reactive fragments increases with the increase of impact velocity, resulting in the decrease of the mass and size of the remaining active fragments. When the collision velocity is low, the perforation of active fragments mainly depends on their own kinetic energy, and the aperture size is also closely related to the diameter of fragments. When the collision velocity is 778 m/s, the mass of fragments participating in the reaction is less, the degree of fragmentation is low, and the residual size is larger than that of 1017 m/s, so the perforation diameter is larger. When the collision velocity is high, more mass of the active fragment participates in the reaction, and the chemical energy released is enough to damage the front target, thus increasing the penetration aperture, which is more reflected in the combined perforation effect of kinetic energy and chemical energy.

Table 2 Image of typical target damage area

表2 典型靶板毁伤区域图片

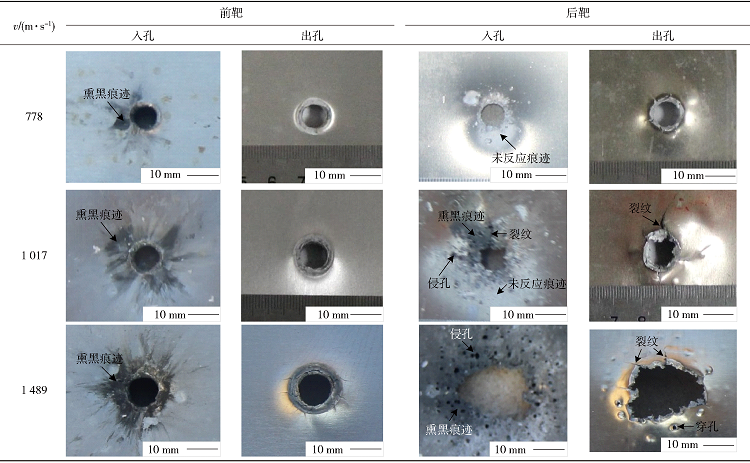Table 3 Experimental results of reactive fragments damage to spacer target

表3 活性破片侵彻间隔靶毁伤面积及穿孔情况实验数据

序号	碰撞速度/ (m·s^-1)	破片截面积/mm²	前靶毁伤面积/mm²	前靶穿孔模式	后靶毁伤面积/mm²	贯穿孔半径/mm	毁伤区半径/mm	后靶穿孔模式
1	778	50.2655	64.3774	冲塞型	50.2359	4.12	7.50	花瓣型
2	780	50.2655	63.2158	冲塞型	50.4183	4.12	7.51	花瓣型
3	1017	50.2655	54.6541	冲塞型	71.2300	5.30	16.00	花瓣型
4	1024	50.2655	55.1223	冲塞型	72.6291	5.32	16.04	花瓣型
5	1489	50.2655	86.5922	冲塞型	332.3194	11.00	31.49	花瓣型
6	1493	50.2655	86.9156	冲塞型	334.4931	11.10	34.51	花瓣型

It can be seen from the rear target damage morphology in Table 2 that the impact velocity has a significant effect on the rear target damage morphology. With the increase of impact velocity, the size of the central penetration hole and the damage area around the penetration hole increase. It is worth noting that with the increase of collision velocity from 778 m/s to 1017 m/s and 1489 m/s, there are no blackening reaction marks, partial blackening reaction marks and global blackening reaction traces in the surrounding damage area, respectively. With the increase of collision velocity, the deformation and uplift degree of the surrounding damage area increase, and the number of intrusion holes increases with the increase of collision velocity. Mechanistically, after the active fragment penetrates the front target, the remaining fragments break up and form a series of fragments of different sizes that continue to collide with the rear target, and form the same main perforation on the rear target as the front target. However, due to the secondary collision, the active fragments are more likely to react at this time, especially with the increase of collision speed, the activation degree of active fragments is higher, and the energy released by the reaction is more, so a large central perforation is formed at the rear target position. At the same time, there is a certain scattering angle after the fragment penetrates the front target, and the fragment with a large scattering angle gradually deviates from the original fragment trajectory under the influence of the lateral velocity and impacts the rear target around the central penetration hole.A series of penetrating or non-penetrating holes are formed, which mainly depends on the mass, speed and activation degree of small fragments. The larger the mass, the higher the speed and the higher the activation degree of fragments, the easier the formation of penetrating holes.

Typical high-speed photographs of reactive fragments penetrating spaced targets at different impact velocities are shown in Figure 4. By further analyzing the influence of the collision velocity on the damage effect, it can be seen from Figure 4 that when the active fragment collides with the front target at different velocities, it produces different degrees of fire at the collision position. Comparing Fig. 4 (a) to Fig. 4 (C), it is found that the brightness of the fire is higher and the range is larger with the increase of the collision speed, which indicates that the activation degree of the active fragments increases with the increase of the collision speed. In the process of subsequent penetration into the front target, the active fragments produce different degrees of bright yellow flame backward, and the brightness and range of the flame increase with the increase of collision velocity. This is due to the fact that the reactive fragments have reacted during the penetration process, and the degree of reaction increases with the increase of the collision velocity, which is consistent with the fact that the jet-like reaction marks left on the front target in Table 2 become more significant with the increase of the collision velocity. Under the impact load, the time from the activation of the internal active elements to the deflagration reaction of the active fragment is the delay time of the active fragment. By comparing and analyzing the time when the bright yellow flame is generated in Fig. 4 (a) to Fig. 4 (C), it can be seen thatIn the process of penetration, the delay time of active fragments decreases with the increase of impact velocity, which is consistent with the increase of activation degree of active fragments when they collide with the target before impact.

Fig.4 Typical high-speed photographs

图4 典型高速摄像

Full size|PPT slide

It can be seen from the firelight after the active fragment penetrates the front target in Fig. 4 that some of the remaining fragments continue to react, forming an approximately ellipsoidal coupling damage zone between the spaced target plates, and at the same time, the expansion area of the coupling damage zone and the firelight brightness increase significantly with the increase of the collision velocity. Further analysis shows that the residual velocity and activation degree of active fragments after penetrating the front target increase with the increase of collision velocity, and the fragments with higher activation degree release more chemical energy during the dispersion process.Therefore, the brightness of the fire is higher, and the chemical reaction is centered on the activated debris, and the movement speed of the debris coupling damage zone with higher residual speed is faster. When the remaining fragments collide with the rear target for the second time, further reaction occurs to release chemical energy, and the amount of chemical energy release increases with the increase of the collision velocity, so that the flame brightness, the action surface, and the flame radial spread distance increase with the increase of the collision velocity. This is also consistent with the increasing trend of the size of the through hole in the center of the rear target and the damage area with the increase of the collision velocity in Table 2.

3 Analysis and discussion

3.1 Behind target debris cloud shaping

Based on the impact reaction characteristics of active fragments, the penetration process of active fragments into the target is shown in Figure 5. The active fragment collides with the front target at a certain initial velocity, and is deformed and broken in the penetration process. When the active fragment penetrates the front target, the broken part is scattered to form a debris cloud behind the target, which continues to move forward and impacts the rear target. When the debris cloud behind the target collides with the rear target, a violent deflagration reaction occurs to release energy^[19]. Under the combined action of kinetic penetration and chemical energy release, the structural damage of the rear target occurs.

Fig.5 Process of reactive fragments penetrating the plate

图5 活性破片侵彻靶板作用过程

Full size|PPT slide

In order to analyze the interaction behavior of active fragment projectile with target, a model of debris cloud formation behind the target was established. Based on the one-dimensional shock wave theory, the target shock pressure p₀ before the collision of active fragments is derived from the conservation of momentum as^[20]

\{\begin{array}{l} p_{0} = \frac{(ρ_{p} c_{p} + ρ_{t} c_{t} + 2 ρ_{p} s_{p} v_{0}) - \sqrt{Δ}}{2 (ρ_{p} s_{p} - ρ_{t} s_{t})} ρ_{t} c_{t} \\ Δ = (ρ_{p} c_{p} + ρ_{t} c_{t} + 2 ρ_{p} s_{p} v_{0})^{2} + \\ 4 ρ_{p} (ρ_{t} s_{t} - ρ_{p} s_{p}) (c_{p} v_{0} + s_{p} v_{0}^{2}) \end{array}

(1)

Where :ρ_p and ρ_t are the active fragment and target plate densities, respectively; C and s are the sound velocity and material coefficient respectively, and the subscripts p and t represent the active fragment and target plate respectively; v₀ is the impact velocity. The c_t and s_t of 2A 12 aluminum are 5328m/s and 1.338 respectively^[19]; The active fragment c_p is 4540m/s, and the active fragment s_p is 1.17 according to the mass interpolation method^[21].

The shock pressure distribution in the active fragment is reduced to a position-dependent exponential function:

p (x) = p_{0} e^{(- δ_{p} x)}

(2)

Where: p (X) is the pressure in the active fragment at X from the collision interface; The δ_p is an empirical constant related to the material properties, and the δ_p of active fragments in this paper is about 0.08663mm^-1^[22]. When the pressure inside the active fragment exceeds the pressure threshold required for it to break, it is considered to break. Therefore, the fragmentation size L_f1 of active fragments can be expressed as

L_{f 1} = ln (\frac{p_{0}}{Y_{c}}) / δ_{p}

(3)

Where :Y_c is the pressure threshold for complete fragmentation of active fragments, which is about 2780 MPa^[13].

After impacting the front target, the shock wave in the active fragment is unloaded by the rarefaction wave, and the size L_f2 can be expressed as^[19]

L_{f 2} = h_{t} \frac{U_{p} (1 + (U_{t} - u_{t}) / C_{t})}{U_{t} (1 - (U_{p} - u_{p}) / C_{p})}

(4)

Where :U_p and U_t are the shock wave velocities in the active fragment and the target plate, respectively; The h_t is the front target thickness; u_p and u_t are the particle velocities of the active fragment and the target plate, respectively; The rarefaction wave velocity C_j can be expressed as^[19]

C_j=U_j{0.49+[(U_j-u_j)/U_j]²}⁰^.⁵

(5)

j=p, t。

Therefore, the real breakage size L_f of active fragments can be expressed as

L_f=min(L_f₁,L_f₂)

(6)

According to the experimental results, in the experimental impact velocity range, the damage mode of active fragments penetrating into the target can be approximated to plugging damage mode. In order to obtain the residual velocity of the fragment after penetrating the front target, the deformation of the fragment and the plug in the penetration process is ignored, and it is assumed that the energy dissipated by the resistance of the target plate during the penetration of the fragment into the front target is E_f1, the shear strain energy when the plug is produced is E_f2, and the kinetic energy of the plug is E_f3. Then according to the conservation of energy

\frac{1}{2} m_{p} v_{0}^{2} = {E_{f}}_{1} + {E_{f}}_{2} + {E_{f}}_{3} + \frac{1}{2} m_{p} v_{r}^{2}

(7)

Where :m_p is the active fragment mass; E_f1, E_f2, E_f3 can be respectively expressed as^[23]

\{\begin{array}{l} E_{f 1} = \frac{A_{p l u g} t_{p l u g}}{6 G_{t}} P_{0}^{2} \\ E_{f 2} = \int_{0}^{t_{}} τ_{u d} A_{s} d x = π D t_{p l u g}^{2} τ_{u d} \\ E_{f 3} = \frac{1}{2} m_{p l u g} v_{r}^{2} \end{array}

(8)

A_plu_g and t_plug are the plug area and plug thickness, G_t is the shear modulus of the target plate, about 28GPa,τ_ud is the dynamic shear fracture strength of the target plate,Generally, it can be expressed as twice the static shear fracture strength τ_u, about 600MPa,A_s is the area of the sheared region, D is the fragment diameter, and m_plug is the plug mass^[24].

According to equations (7) and (8), the residual velocity v_r of the reactive fragment after penetrating the front target can be expressed as

v_{r} = \sqrt{(m_{p} v_{0}^{2} - 2 (\frac{A_{p l u g} t_{p l u g}}{6 G_{t}} p_{0}^{2} + π D t_{p l u g}^{2} τ_{u d})) / (m_{p} + m_{p l u g})}

(9)

According to [25], after the fragment penetrates the front target, the average fragment size s_a of the broken part can be expressed as

s_{a} = \sqrt{\frac{1.2 Y}{ρ_{p} (L_{f} (v_{0} + v_{r}) / 2 L^{2})^{2}}}

(10)

In the formula, Y is the yield strength of the active fragment; L is the length of active fragment.

Assuming that the fragments after active fragment breakage are spherical, the total number of fragments N₀ can be approximated as

N_{0} = 6 \frac{L_{f}}{L_{0}} \cdot \frac{m_{p}}{π s_{a}^{3} ρ_{p}}

(11)

According to the experimental damage results and the empirical formula, the maximum scattering angle θ_max of reactive fragments can be expressed as^[26]

θ_{m a x} = 68.3465 (\frac{v_{0}}{U_{t}}) - 6.8961

(12)

The fragments of the debris cloud behind the target can be approximately considered to be distributed in a truncated cone. For ease of calculation, it is assumed that the opposite direction of the debris vector in the debris cloud meets at the center of the front target. According to the divergence angle of the spatial position of the debris, the number of debris contained in the divergence angle, N_θ, can be expressed as^[27]

N_{θ} = \frac{tan θ}{tan θ_{max}} N_{0}

(13)

According to equation (13), the number of fragments N_θi within the scattering angle [θ, θ + dθ] can be expressed as

N_{θ i} = \frac{tan (θ + d θ) - tan θ}{tan θ_{max}} N_{0}

(14)

It is generally believed that the velocity of debris in the debris cloud is distributed in a gradient, and the velocity of debris in the range of [θ, θ + dθ] is the same. Where the fragment velocity v_θi in the range of [θ, θ + dθ] scattering angle can be expressed as^[27]

v_{θ i} = \frac{v_{r} cos (1.94 θ_{i})}{cos θ_{i}}

(15)

According to formula (12), formula (14) and formula (15), the theoretical model of debris cloud formation of metal-based active fragments is established, and the evolution law of debris cloud after active fragments penetrate the front target at different collision velocities is obtained through the theoretical model, as shown in Figure 6. It can be seen from Fig. 6 that the active fragment forms an ellipsoidal debris cloud after penetrating the front target. With the increase of the evolution time t, the debris cloud expands along the axial and radial directions, and the expansion area increases with the increase of the collision velocity at the same time, which is consistent with the expansion law of the coupling damage area in Fig. 4. This shows that with the increase of collision velocity, the debris cloud has a stronger expansion ability in the evolution process, a larger damage volume of active fragments, and a larger damage area when impacting the rear target.

Fig.6 Evolution of post-target debris cloud with different impact velocities

图6 不同碰撞速度活性破片靶后碎片云演化规律

Full size|PPT slide

3.2 Rear target damage effect

According to the analysis of the experimental results in Table 2, there are two parts of reacted and unreacted fragments when the active fragments collide with the target at the collision velocity of 1017m/s. According to the intersection of the two parts, the mass of the reactive fragment and its corresponding critical pressure are obtained by combining formula (1), formula (2) and formula (13), which is approximated as the critical reaction pressure A_r of the reactive fragment, which is about 8.16 GPa. Therefore, the activation size L_a₁ when the active fragment collides with the front target can be expressed as

{L_{a}}_{1} = ln (\frac{p_{0}}{A_{r}}) / δ_{p}

(16)

Combined with equation (6), the real activation size L_a of the active fragment is

L_a=min(L_f₁,L_f₂,L_a₁)

(17)

The curves of active fragment breaking size, rarefaction wave unloading size and activation size with collision velocity are shown in Fig. 7. It can be seen from Fig. 7 that rarefaction wave unloading does not occur at collision velocities from 200 to 1500 m/s due to the small size of active fragments. The initial crushing velocity of the active fragment is 241m/s, and the complete crushing velocity is 467m/s, which indicates that the active fragment has been completely crushed at the lowest velocity of 778m/s in this experiment, and has the prerequisite for reaction^[3]. The initial activation velocity of the active fragment is 665m/s, and the complete activation velocity is 1236m/s, which indicates that when the collision velocity is greater than 1236m/S, the active fragment has the condition of complete reaction energy release, and if the collision velocity continues to increase, the reaction energy release of the active fragment when impacting the rear target will not increase.

Fig.7 Fragmentation, activation and sparse wave unloading size of reactive fragments

图7 活性破片破碎尺寸、激活尺寸和稀疏波卸载尺寸

Full size|PPT slide

According to equations (12), (14) and (15), the total kinetic energy E_k of the active fragment cloud can be expressed as

E_{k} = \sum \frac{N_{θ i}}{2 N_{0}} m_{p} v_{θ i}^{2}

(18)

The activation degree of active fragments is obtained according to the real activation size of active fragments in formula (17), which is used to characterize the total chemical energy of active fragment cloud. Assuming that the energy released by the reaction of the activated part is the same, independent of the part exceeding the activation pressure threshold, the activation degree A_d of the active fragment cloud can be expressed as

A_{d} = \frac{min (L_{a}, L)}{L}

(19)

The total kinetic energy and activation degree of the active fragment cloud as a function of collision velocity are shown in Figure 8. It can be seen from Fig. 8 that when the collision velocity is greater than 665 m/s, the activation degree of the debris cloud behind the target, that is, the chemical energy contained in the debris cloud, increases with the increase of the collision velocity, and reaches 1 when the collision velocity reaches 1236 m/s. At this time, it can be approximately considered that the active fragments have been fully activated during the penetration of the front target, and the chemical energy in the debris cloud can be completely released when colliding with the rear target. With the increase of experimental collision velocity, the activation degree of active fragments is 0.247, 0.678 and 1.000, respectively. At the same time, according to formula (9), the ballistic limit velocity of active fragments penetrating 6mm thick 2A12 aluminum target is about 718 m/s. When the impact velocity is greater than 718 m/s, the kinetic energy of the debris cloud behind the target increases with the increase of the impact velocity. With the increase of the experimental collision velocity, the kinetic energy in the active fragment cloud is 70.6 J, 399.1 J and 1265.6 J, respectively. Therefore, when the collision velocity is 718 ~ 1236m/s, the chemical energy and kinetic energy in the active fragment cloud increase logarithmically and exponentially with the increase of the collision velocity, respectively.When the collision velocity is greater than 1236m/s, only the kinetic energy in the active fragment cloud increases exponentially.

Fig.8 Relationship between the total kinetic energy and activation degree

图8 活性破片碎片云总动能和激活程度与碰撞速度关系

Full size|PPT slide

The schematic diagram of debris cloud acting on the target plate behind the active fragment target is shown in Fig. 9. The damage mode of debris cloud to the rear target is mainly the central penetration mode and the debris impact mode. Fig. 9 (a) is a schematic diagram of the inert debris cloud acting on the target plate. The inert debris cloud acting on the target plate is only affected by the penetration of the debris cloud kinetic energy, and the central penetration aperture is affected by the debris cloud kinetic energy, which increases with the debris scattering angle.According to formula (15), the kinetic energy of the unit fragment decreases, and the fragment requires more energy to penetrate the target obliquely, so it is gradually unable to penetrate the target, and only small pits and holes are formed. Fig. 9 (B) is a schematic diagram of the active debris cloud acting on the target plate. The active debris cloud acting on the target plate is subjected to the combined action of debris kinetic energy penetration and chemical energy release. At the critical penetration position in the inert mode, the active debris reacts to release energy, which increases the critical penetration aperture. The active fragments release energy during the penetration process, and the greater the kinetic energy of the fragments, the stronger the penetration ability, and the more obvious the reaming phenomenon caused by the chemical energy released by the reaction.

Fig.9 Schematic diagram of target plate acted by debris cloud

图9 碎片云作用靶板示意图

Full size|PPT slide

It can be seen from Fig. 9 that the kinetic energy and chemical energy release of the active debris cloud acting on the target have an important influence on the damage effect of the target. Therefore, according to equations (11), (14), (15) and (19), the unit fragment kinetic energy and reaction mass of the debris cloud within a certain dispersion angle are obtained. Where the unit debris kinetic energy distribution E_e_i of the debris cloud can be expressed as

{E_{e}}_{i} = \frac{m_{p} v_{θ i}^{2}}{2 N_{0}}

(20)

The unit fragment reaction mass distribution m_a_i of the debris cloud can be expressed as

{m_{a}}_{i} = {\begin{cases} \frac{A_{d} m_{p}}{N_{0}}, & θ \leq arctan (A_{d} tan θ_{max}) \\ 0, & θ > arctan (A_{d} tan θ_{max}) \end{cases}

(21)

According to equations (20) and (21), the kinetic energy per unit fragment and the reaction mass at the position of the target plate penetrating the aperture at different collision velocities are shown in Figure 10. It can be seen from Fig. 10 that at the collision velocity of 778 m/s, the kinetic energy per unit fragment at the perforation position is about 0.049 J, and due to the low degree of activation at this collision velocity, the fragment at this position does not react, so the reaction mass per unit fragment is 0 mg, which is consistent with the unreacted trace on the rear target in Table 2. At the impact velocity of 1 017 m/s, the unit fragment kinetic energy at the perforation position is about 0. 034 J, which is lower than that at the impact velocity of 778 m/s, but the unit fragment reaction mass is 0. 47 mg, and the target plate is subjected to kinetic energy and chemical energy coupling at this position. At the collision velocity of 1 489 m/s, the unit fragment kinetic energy at the penetration hole is about 0. 042 J, and the unit fragment reaction mass is 0. 124 mg, which is higher than that at 1 017 m/s, so the unit fragment reaction mass required to penetrate the target plate is less.

Fig.10 Unit debris kinetic energy and reaction mass at the position of rear target through aperture at different impact velocities

图10 不同碰撞速度下后靶贯穿孔径位置处单位碎片动能与反应质量

Full size|PPT slide

4 Conclusion

In this paper, the formation and damage mechanism of debris cloud behind metal-based active fragment target are studied by ballistic gun experiment, and the evolution law of debris cloud behind target and the influence law of collision velocity on target damage effect are obtained. The main conclusions are as follows:

The main results are as follows: 1) The damage mechanism of metal-based reactive fragments to spaced targets is revealed. Before penetration, the target is perforated mainly by the coupling of fragment kinetic energy and reaction chemical energy, and the perforation decreases first and then increases with the increase of impact velocity.The rear target is mainly damaged by the debris cloud formed after the active fragments penetrate the front target, and the damage mode is mainly the central penetration mode and the debris impact mode, and the aperture of the central penetration area is positively correlated with the impact velocity.

2) The interaction behavior of metal-based active fragments with spacer targets was analyzed, and the variation of activation degree with collision velocity was obtained, which was positively correlated with collision velocity.

3) The theoretical model of debris cloud formation behind the metal matrix active fragment target is established, and the evolution law of debris cloud behind the target is obtained. The aperture of the penetration zone behind the target is coupled by the kinetic energy of debris cloud and the energy released by the reaction. At the critical penetration position, the energy released by the fragment reaction is negatively correlated with the kinetic energy.

References

Publishing order | Descend order by publishing year | Descend order by cited within

[1]

肖艳文, 徐峰悦, 余庆波, 等. 类钢密度活性材料弹丸撞击铝靶行为实验研究[J]. 兵工学报, 2016, 37(6):1016-1022.

https://doi.org/10.3969/j.issn.1000-1093.2016.06.007

Abstract

采用弹道碰撞实验对类钢密度冷压成型和烧结硬化聚四氟乙烯/铝/钨系活性材料弹丸撞击铝靶行为进行了研究。基于圆柱形活性材料弹丸正碰撞不同厚度2A12硬铝靶的弹道极限速度、穿孔破坏模式及平均穿孔尺寸实验结果，结合THOR侵彻方程，得到活性材料弹丸正碰撞铝靶的弹道极限速度半经验关系，并分析铝靶厚度对活性材料弹丸相对于钢弹丸侵彻行为及性能的影响。从活性材料内部压力分布、靶板背面稀疏波卸载效应和活性材料激活响应点火时间等角度，分析和讨论了活性材料弹丸化学响应行为对侵彻性能的影响机理。分析结果表明，随着靶板厚度的增大，活性材料激活率和侵孔内爆燃压力随之提高，从而导致侵彻末端爆裂穿孔能力的显著增强。

XIAO

Y W

, XU

F Y

, QU

Q B

, et al. Experimental research on behavior of active material projectile with steel-like density impacting aluminum target[J]. Acta Armamentarii, 2016, 37(6):1016-1022. (in Chinese)

Cited in this article [1]

[2]

王海福, 郑元枫, 余庆波, 等. 活性破片引燃航空煤油实验研究[J]. 兵工学报, 2012, 33(9):1148-1152.

Abstract

进行弹道炮发射实验，研究活性破片和钨合金破片作用模拟油箱和引燃航空煤油问题。实验结果表明：在1 080 m/s着速下，活性破片击穿10 mm厚LY12铝靶后能可靠引燃航空煤油，而同质量钨合金破片以1 643 m/s的速度命中油箱，只造成油箱穿孔及漏油，未能引燃燃油；常温状态下，活性破片较钨合金破片具有更强的引燃航空煤油能力，活性破片的内爆作用和化学能释放是造成油箱结构严重破坏和燃油燃烧的主要原因。进行弹道炮发射实验，研究活性破片和钨合金破片作用模拟油箱和引燃航空煤油问题。实验结果表明：在1 080 m/s着速下，活性破片击穿10 mm厚LY12铝靶后能可靠引燃航空煤油，而同质量钨合金破片以1 643 m/s的速度命中油箱，只造成油箱穿孔及漏油，未能引燃燃油；常温状态下，活性破片较钨合金破片具有更强的引燃航空煤油能力，活性破片的内爆作用和化学能释放是造成油箱结构严重破坏和燃油燃烧的主要原因。

WANG

H F

, ZHENG

Y F

, YU

Q B

, et al. Experimental research on igniting the aviation kerosene by reactive fragment[J]. Acta Armamentarii, 2012, 33(9):1148-1152. (in Chinese)

Cited in this article [1]

[3]	AMES R. Energy release characteristics of impact initiated energetic materials[J]. MRS Online Proceeding Library Archive, 2005, 896(3):321-333. Cited in this article [2]

[4]	赵宏伟, 余庆波, 邓斌, 等. 活性破片终点毁伤威力试验研究[J]. 北京理工大学学报, 2020, 40(4):375-381. ZHAO H W, YU Q B, DENG B, et al. Experimental study on terminal demolition lethality of reactive fragments[J]. Transactions of Beijing Institute of Technology, 2020, 40(4):375-381. (in Chinese) Cited in this article [1]

[5]	陈思源, 王在成, 仝远. PTFE基含能破片的能量释放特性研究[J]. 北京理工大学学报, 2017, 37(增刊2):62-65. CHEN S Y, WANG Z C, TONG Y. Energy release characteristics of PTFE-based energetic materials[J]. Transactions of Beijing Institute of Technology, 2017, 37(S2):62-65. (in Chinese) Cited in this article [1]

[6]

王在成, 李姝妍, 姜春兰, 等. 高Al含量PTFE基材料爆炸冲击压缩特性及反应行为[J]. 北京理工大学学报, 2021, 41(4):356-363.

WANG

Z C

, LI

S Y

, JIANG

C L

, et al. Impact compression characteristics and reaction behavior of high Al content PTFE based materials under explosive loading[J]. Transactions of Beijing Institute of Technology, 2021, 41(4):356-363. (in Chinese)

Cited in this article [1]

[7]

李鑫, 王伟力, 梁争峰, 等. 复合结构活性破片对双层靶标毁伤效应[J]. 兵工学报, 2021, 42(4):764-772.

, WANG

W L

, LIANG

Z F

, et al. Damage effect of composite structural reactive fragments on double-layer targets[J]. Acta Armamentarii, 2021, 42(4):764-772. (in Chinese)

https://doi.org/10.3969/j.issn.1000-1093.2021.04.010

Cited in this article [1] Abstract

It is of great significance to study the damage power of reactive fragments which can withstand the loading of explosive. The damage effect of Al/PTFE composite structural reactive fragments on the different types of double-layer targets is studied through the loading experiment with 14.5 mm ballistic gun. The empiricial formulas of penetration diameter in front layer plate and expanding perforation area on rear layer plate were established using multiple regression analysis. The results show that the penetration diameter in front layer steel or aluminum plate increases with the rising of impact velocity and target thickness in the range of 800-1 400 m/s. The penetration diameters in the steel, aluminum and carbon fiber composite plates are 1.25-1.62 times, 1.08-1.42 times and 1.13 times of fragment diameter, respectively. Expanding and tearing damages are caused to the rear layer aluminum plate or the carbon fiber composite plate by the ractive fragments. The expanding perforation area increases with the increase in front layer target strength and impact velocity, and the delamination of carbon fiber and resin matrix for the back of carbon fiber composite plate should be considered in damage assessment. The empiricial formulas were proved to be accurate and reliable, and the relative error was controlled within 5% through experiment.

[8]	徐光泽, 张良, 张兴高, 等. 活性破片侵彻Q235钢靶穿燃后效实验研究[J]. 火工品, 2021(2):19-22. XU G Z, ZHANG L, ZHANG X G, et al. Study on aftereffect of penetrating Q235 stell by reactive fragment[J]. Initiators and Pyrotechnics, 2021(2):19-22. (in Chinese) Cited in this article [1]

[9]	GRUDZA M, FLIS W J, LAM H L, et al. Reactive material structures[R]. King of Prussia, PA, US: DE Technologies Inc, 2014. Cited in this article [1]

[10]	陈进, 梁争峰, 周涛. 金属型活性破片战斗部技术研究进展[J]. 飞航导弹, 2019(4):89-95. CHEN J, LIANG Z F, ZHOU T. Research progress of metal type active fragments warhead technology[J]. Maneuverable Missile, 2019(4):89-95. (in Chinese) Cited in this article [1]

[11]	曹召勋, 王军, 徐永东, 等. Al-Ni活性金属材料力学性能及其释能行为研究[J]. 兵器材料科学与工程, 2018, 41(5):90-96. CAO Z X, WANG J, XU Y D, et al. Study on mechanical properties and energy release behavior of active metal materials[J]. Ordnance Material Science and Engineering, 2018, 14(5):90-96. (in Chinese) Cited in this article [1]

[12]

陈元建, 陈进, 王军, 等. Al-Ni-W活性材料动态力学行为及其释能特性[J]. 兵器材料科学与工程, 2018, 41(4):71-75.

CHEN

Y J

, CHEN

, WANG

, et al. Dynamic mechanical behavior and energy release characteristics of Al-Ni-W active materials[J]. Ordnance Material Science and Engineering, 2018, 41(4):71-75. (in Chinese)

Cited in this article [1]

[13]

刘晓俊, 任会兰, 宁建国. Zr-W多功能含能结构材料的制备及动态压缩特性[J]. 复合材料学报, 2016, 33(10):2297-2303.

LIU

X J

, REN

H L

, NING

J G

. Preparation and dynamic compression properties of Zr-W multifunctional energetic structural materials[J]. Journal of Composite Materials, 2016, 33(10):2297-2303. (in Chinese)

Cited in this article [2]

[14]	陈伟, 赵文天, 王健. 钨锆合金破片毁伤过程研究[J]. 兵器材料科学与工程, 2009, 32(2):108-111. CHEN W, ZHAO W T, WANG J, et al. Research of damaging process for W-Zr alloy fragment[J]. Ordnance material science and engineering, 2009, 32(2):108-111. (in Chinese) Cited in this article [1]

[15]	王璐瑶, 蒋建伟, 李梅. 钨锆铪合金活性破片对间隔靶耦合毁伤特性[J]. 兵工学报, 2020, 41(增刊2):144-148. WANG L Y, JIANG J W, LI M. Experimental research on damage behavior of W-Zr-Hf alloy fragment to spaced targets[J]. Acta Armamentarii, 2020, 41(S2):144-148. (in Chinese) Cited in this article [1]

[16]

王璐瑶, 蒋建伟, 李梅, 等. 钨锆铪活性合金破片冲击释能行为实验研究[J]. 兵工学报, 2019, 40(8):1603-1610.

https://doi.org/10.3969/j.issn.1000-1093.2019.08.007

Abstract

为研究活性合金材料的冲击释能行为，采用弹道枪驱动一种高强度、高密度的钨锆铪活性合金破片，使其以不同着速撞击Q235钢靶，通过观察靶板穿孔模式和靶后冲击释能火光区的高速摄影图像，提出合金类破片冲击释能的3阶段模型，即冲击激活阶段、自蔓延释能阶段、自激活阶段。结合应力波、热应力理论和冲击温升方程，获得该破片能量的激活门限、能量完全释放的临界条件、释能火光区的最大尺寸及其靶后有效毁伤距离。研究结果表明：钨锆铪活性合金破片不仅具有类似惰性破片的动能毁伤能力，而且在穿靶前活性能耗极小，活性能量在穿靶后毫秒量级内完全释放；破片活性能量被完全激活前，释能火光区的最大毁伤容积和有效毁伤距离分别随破片着速的提高呈指数和线性增长趋势。

WANG

L Y

, JIANG

J W

, LI

, et al. Experimental research on energy release behavior of W/Zr/Hf alloy fragment[J]. Acta Armamentarii, 2019, 40(8):1603-1610. (in Chinese)

https://doi.org/10.3969/j.issn.1000-1093.2019.08.007

Cited in this article [1] Abstract

To investigate the energy release behavior of reactive material under impacting conditions, a high-strength and high-density tungsten-zirconium-hafnium active alloy fragment is driven to impact the Q235 steel target at different speeds by a ballistic gun. A three-stage, including shock activation, self-propagation energy release, and secondary self-activation, model of impact-release energy of alloy fragments is proposed by observing the perforation pattern of steel target and the high-speed photographic image of the post-target impact-release fire zone.The activation threshold of fragment energy, the critical condition of complete energy release, the maximum size of energy release flare region and the effective damage distance behind the target were obtained by applying stress wave, thermal stress theory and shock temperature rise equation. The results show that the tungsten-zirconium-hafnium active alloy fragment not only has the kinetic energy damage ability similar to the inert fragment, but also has a little active energy consumption before the target is penetrated, and the active energy is completely released in a millisecond time regime after penetrating into the target. Before the fragmentation active energy is fully activated, the maximum damage volume and effective damage distance in the release energy flare region increase exponentially and linearly with the increase in fragmentation speed. Key

[17]	李梅, 王璐瑶, 蒋建伟. 典型反应性合金冲击释能量及耦合毁伤能力对比[J]. 兵工学报, 2021, 42(9):1867-1876. LI M, WANG L Y, JIANG J W. Comparisonof released energy and coupling damageability of typical reactive alloy under high-speed impact on target[J]. Acta Armamentarii, 2021, 42(9):1867-1876. (in Chinese) Cited in this article [1]

[18]

卢冠成, 曹康, 耿宝群, 等. 活性破片爆裂毁伤后效快速图像识别方法[C]//第12届全国爆炸力学学术会议论文集. 桐乡: 中国力学学会, 2018.

G C

, CAO

, GENG

B Q

, et al. Fast image recognition method for aftereffect of reactive fragment explosion[C]//Proceedings of the 12th national conference on explosive mechanics. Tongxiang: Chinese society of mechanics, 2018. (in Chinese)

Cited in this article [1]

[19]

F Y

, YU

Q B

, ZHENG

Y F

, et al. Damage effect of double-spaced aluminum plates by reactive material projectile impact[J]. International Journal of Impact Engineering, 2017, 104:13-20.

https://doi.org/10.1016/j.ijimpeng.2017.01.023

https://linkinghub.elsevier.com/retrieve/pii/S0734743X16306698

Cited in this article [4]

[20]

Q B

, ZHANG

J H

, ZHAO

H W

, et al. Behind-plate overpressure effect of steel-encased reactive material projectile impacting thin aluminum plate[J]. Defence Technology, 2021, 18(5):723-734.

https://doi.org/10.1016/j.dt.2021.03.022

https://linkinghub.elsevier.com/retrieve/pii/S2214914721000489

Cited in this article [1]

[21]

SWAN

C C

, KOSAKA

. Voigt-reuss topology optimization for structures with nonlinear material behaviors[J]. International Journal for Numerical Methods in Engineering, 1997, 40(20):3785-3814.

https://doi.org/10.1002/(ISSN)1097-0207

http://doi.wiley.com/10.1002/%28ISSN%291097-0207

Cited in this article [1]

[22]	刘沫言. 带壳装药的破片撞击和冲击波感度研究[D]. 沈阳: 沈阳理工大学, 2020. LIU M Y. Study on fragment impact and shock wave sensitivity of shell charge[D]. Shenyang: Shenyang University of Technology, 2020. (in Chinese) Cited in this article [1]

[23]

NIKNEJAD

, ZAREEI

. Ballistic limit velocity of empty rectangular metal columns under a blunt projectile penetration[J]. The Journal of Defense Modeling and Simulation: Application, Methodology, Technology, 2016, 13(1):119-131.

https://doi.org/10.1177/1548512915590611

http://journals.sagepub.com/doi/10.1177/1548512915590611

Cited in this article [1] Abstract

This paper studies the ballistic limit velocity of empty rectangular columns under a blunt projectile penetration, theoretically and numerically. The deformation mechanism of the empty column is considered as plugging. The projectile is assumed to be a flat-ended cylinder without deformation. A new theoretical model of deformation is introduced, and based on the energy method, a theoretical formula is derived to estimate the ballistic limit velocity of the process. Numerical simulations of the penetration process of a blunt solid cylinder into the empty metal columns are performed using the LS-DYNA explicit solver. Different empty columns are used as the targets, and these columns are made from Weldox 460E steel and have different wall thicknesses (6, 7, 8 and 9 mm) and internal column edge lengths (60, 70, 80 and 90 mm). To validate the derived relation for the ballistic limit velocity, the results of the numerical simulations are compared with the corresponding theoretical predictions. Finally, the effects of some geometrical characteristics such as projectile diameter and mass on the perforation process and the ballistic limit velocity are discussed.

[24]

LIANG

C C

, YANG

M F

, WU

P W

, et al. Resistant performance of perforation of multi-layered targets using an estimation[J]. Ocean Engineering, 2005; 32(3/4):441-468.

https://doi.org/10.1016/j.oceaneng.2004.05.009

https://linkinghub.elsevier.com/retrieve/pii/S002980180400191X

Cited in this article [1]

[25]

PEDERSEN

, BLESS

. Behind-armor debris from the impact of hypervelocity tungsten penetrators[J]. International Journal of Impact Engineering, 2006, 33(1-12):605-614.

https://doi.org/10.1016/j.ijimpeng.2006.09.007

https://linkinghub.elsevier.com/retrieve/pii/S0734743X06001242

Cited in this article [1]

[26]	MAYSELESS M, SELA N, STILP A J, et al. Behind the armor debris distribution function[C]//Proceedings of 13th International Symposium on Ballistics. Stockholm, Sweden: International Ballistics Society, 1992:77-85. Cited in this article [1]

[27]	李向东, 杜忠华. 目标易损性[M]. 北京: 北京理工大学出版社, 2013. LI X D, DU Z H. Target vulnerability[M]. Beijing: Beijing University of Technology Press, 2013. (in Chinese) Cited in this article [2]

Accesses

Citation

Detail

Sections

Recommended

Abstract
Key words
QR code of this article
Cite this article
0 Introduction
1 Ballistic gun test method
1.1 Experimental sample
Fig.1 Reactive fragments and quasi-static compressive stress-strain curves
Table 1 Mechanical properties of reactive fragments
1.2 Experimental target
Fig.2 Experimental target and target structure schematic
1.3 Experimental principle
Fig.3 Schematic and image of the experimental test system
2 Experimental result
Table 2 Image of typical target damage area
Table 3 Experimental results of reactive fragments damage to spacer target
Fig.4 Typical high-speed photographs
3 Analysis and discussion
3.1 Behind target debris cloud shaping
Fig.5 Process of reactive fragments penetrating the plate
Fig.6 Evolution of post-target debris cloud with different impact velocities
3.2 Rear target damage effect
Fig.7 Fragmentation, activation and sparse wave unloading size of reactive fragments
Fig.8 Relationship between the total kinetic energy and activation degree
Fig.9 Schematic diagram of target plate acted by debris cloud
Fig.10 Unit debris kinetic energy and reaction mass at the position of rear target through aperture at different impact velocities
4 Conclusion
References

Received	Published
2022-04-06	2023-09-06
Just Accepted Date	Issue Date
2022-07-06	2023-08-30

Please choose a citation manager

Content to export

Abstract

Key words

QR code of this article

Cite this article

0 Introduction

1 Ballistic gun test method

1.1 Experimental sample

Fig.1 Reactive fragments and quasi-static compressive stress-strain curves

Table 1 Mechanical properties of reactive fragments

1.2 Experimental target

Fig.2 Experimental target and target structure schematic

1.3 Experimental principle

Fig.3 Schematic and image of the experimental test system

2 Experimental result

Table 2 Image of typical target damage area

Table 3 Experimental results of reactive fragments damage to spacer target

Fig.4 Typical high-speed photographs

3 Analysis and discussion

3.1 Behind target debris cloud shaping

Fig.5 Process of reactive fragments penetrating the plate

Fig.6 Evolution of post-target debris cloud with different impact velocities

3.2 Rear target damage effect

Fig.7 Fragmentation, activation and sparse wave unloading size of reactive fragments

Fig.8 Relationship between the total kinetic energy and activation degree

Fig.9 Schematic diagram of target plate acted by debris cloud

Fig.10 Unit debris kinetic energy and reaction mass at the position of rear target through aperture at different impact velocities

4 Conclusion

{{custom_sec.title}}

{{custom_sec.title}}

References

{{custom_fnGroup.title_en}}

Footnotes

模态框（Modal）标题

Please choose a citation manager

Content to export

Abstract

Key words

QR code of this article

Cite this article

0 Introduction

1 Ballistic gun test method

1.1 Experimental sample

Fig.1 Reactive fragments and quasi-static compressive stress-strain curves

Table 1 Mechanical properties of reactive fragments

1.2 Experimental target

Fig.2 Experimental target and target structure schematic

1.3 Experimental principle

Fig.3 Schematic and image of the experimental test system

2 Experimental result

Table 2 Image of typical target damage area

Table 3 Experimental results of reactive fragments damage to spacer target

Fig.4 Typical high-speed photographs

3 Analysis and discussion

3.1 Behind target debris cloud shaping

Fig.5 Process of reactive fragments penetrating the plate

Fig.6 Evolution of post-target debris cloud with different impact velocities

3.2 Rear target damage effect

Fig.7 Fragmentation, activation and sparse wave unloading size of reactive fragments

Fig.8 Relationship between the total kinetic energy and activation degree

Fig.9 Schematic diagram of target plate acted by debris cloud

Fig.10 Unit debris kinetic energy and reaction mass at the position of rear target through aperture at different impact velocities

4 Conclusion

{{custom_sec.title}}

{{custom_sec.title}}

References

{{custom_fnGroup.title_en}}

Footnotes